|
|
This 1-year Master's program prepares specialists for financial sector, banking and insurance industries in quantitative methods of risk assessment and hedging. The program provides knowledge and skills in two major areas - mathematics of derivative pricing and actuarial (insurance) mathematics. Sound knowledge and interest in mathematics are advantageous to succeeding in this Master's program. |
|
|
|
|
Detailed information about the admission process and necessary documents are available on the web page of the University of Tartu together with a printable brochure. |
|
|
Practical information for international students can be found here. |

|
Tartu is Estonia's second largest city of about 100 000 people. It is famous for its intellectual atmosphere and University spirit as one out of every five citizens of Tartu are students and many more work or teach at the Univesity. |
|
|
|
|
Computational Finance |
| In order to use mathematics for making sound financial decisions, one has to be able to select a suitable market model, to calibrate the model and to use it for computing various numerical quantities (prices of options, hedging parameters etc). Since new and better models are introduced very often, it is not enough to learn to use a fixed set of methods for some concrete applications. The goal of this course is to familiarize the students with the process of deriving appropriate equations from various market models and with the basic techniques of deriving numerical methods for those equations together with giving hands-on experience in implementing the methods effectively in a high level language; the questions of model calibration are also addressed. |
|
Professor Kalev Pärna, Ph.D., Program Manager "In Tartu, we teach the essence of modern financial and insurance mathematics necessary for proper handling of various risks faced by financial enterprises. Our graduates work in local companies and elsewhere, including Wall Street." |

|
|
|
Life Insurance Mathematics |
| What determines the premiums of a life-insurance contract? Clearly the insurance company has to take into account the age, occupation, sex and even habits of the customer and use a model of lifetime. In that sense each contract should be unique. However cash reserves are also required by certain regulations and thus the insurance company must also view its clients as a whole. The big picture is presented in this course. |
|
|
Martingales |
| Martingales are a fundamental tool in many fields of applied probability. The course covers the fundamentals of martingales with examples arising in the financial context. Starting with discrete time martingales, it also covers some basics of continuous time martingales. Ito calculus as well as applications to option pricing thery are discussed. |

|
Associate Professor Raul Kangro, Ph.D. "The ability to derive, implement and use correctly numerical procedures for computing various financial quantities is crucial for being successful in the field of quantitative finance. The computer labs of several courses help to develop those skills." |
|
|
Models of Financial Mathematics |
| Modelling financial market requires several assumptions which are discussed in the first part of the course. The second part introduces scenario based models with discrete time, binomial models and Black-Scholes models, all applicable for option pricing. |
|
|
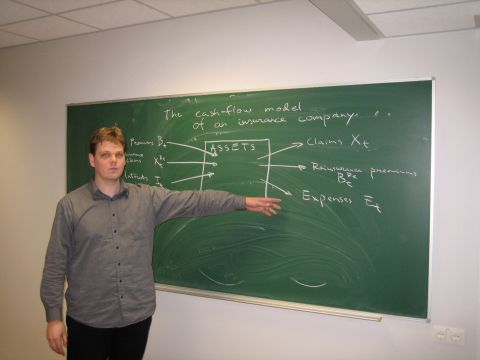
Non-Life Insurance Mathematics |
| Most people have bought or will buy at least one non-life insurance policy (home insurance, car insurance, etc) in their life, which means they are involved with the non-life insurance business as customers. This course gives another point of view to insurance business: through the eyes of an insurance company. We will study what is the reason of several rules and regulations, by what principles the insurance premiums and compensations are calculated and many more topics involving the internal life of an insurance company. |
|
Senior Researcher Meelis Käärik, Ph.D. "Knowing the mathematical background of finance and insurance is essential if you plan your career in the area, but it can also just help you make better choices when buying an insurance policy or stock options." |
|
|
|
Risk Theory |
| Risk is a possibility that an unfavorable event occurs. However, risk is also an opportunity! In order to earn more than by just keeping money on the bank account, one has to take a risk, smaller or larger. Insurance companies take risks by selling insurance policies, which can sometimes lead to huge payouts or even bankruptcy. A classical problem in risk theory what is the ruin probability of an insurance company is the first topic of the course. Risk measures in modern portfolio theory are also covered, including Markowitz model and capital asset pricing model (CAPM). Ubiquitous Value at Risk (VaR) methodology for measuring financial risks, together with the concept of coherent risk measures, are considered. |
|
|
Simulation Methods in Financial Mathematics |
| Very often one is interested in computing values of quantities (like option prices) than can be expressed as expected value of some random variable. A very popular method that can be applied in such cases is the Monte-Carlo method that corresponds to simulating the behavior of the random variable (generating independent samples of the variable) and computing the average of the result. This course discusses the possibilities of using the method for solving problems in mathematical finance and, more importantly, some methods and tricks that can be used to speed up the convergence of the method |
|
|
|
Artur Sepp, Associate, Merrill Lynch (New York) "The Master's program in Financial and Actuarial Mathematics at the University of Tartu provided me with a solid basis on how to use mathematical methods to solve some practical problems in Finance. Importantly, the enthusiastic and helpful faculty has leveraged my knowledge base and expertise." Read more |

|
|
|

|
Meet local and foreign students in an active learning environment. Discuss and discover together while making contacts for the future in the process. WELCOME TO TARTU! |